Concept of Median for group data
A Mathematics teacher of grade 9 took a surprise test (out of 100). The result of test of 50 students is given below:
13, 26, 33, 18, 27, 2, 33, 22, 12, 24, 35, 99, 56, 31, 63, 89, 86 30, 14, 22, 16, 16, 11, 8, 11, 30, 22, 69, 73, 88, 36, 55, 49, 34 83, 4, 17, 7, 8, 75, 13, 23, 3, 1, 3, 1, 11, 4, 12, 22
1. How do you check the performance of a class in a Mathematics Test? Share and discuss possible methods in groups.
2. What are possible reasons behind the low performance of many students? How can we
support them?
3. Find the mean marks of the students.
4. Which method do you prefer in finding mean marks of students: Using raw data, discrete
data or grouped data?
5. What does arithmetic mean tell us about the given data? Do you agree with the result? Why
or why not?
Now, try to find the median of the data.
What challenges do you face in finding the median of the data? Share and discuss possible
solutions.
What arithmetic median tells us about the given data? Do you agree with the result? Why or why
not?
Now, try to find the median of the given data using the frequency distribution table.
What challenges do you face in finding the median of the data? Share and discuss possible
solutions.
Now, try to find the median of the given data using a group frequency distribution table.
Think and discuss with your group about the possible methods to find the median of the group
data.
NOTE
Group frequency distribution table
Step 1:
INFORMATION :
Cumulative frequency is the sum of the class and all classes below it in a frequency
distribution.
Share and discuss: What are the possible reasons behind finding the Cumulative frequency of
all the classes?
NOTE :
The median is the middle value of all the observations. So, to find the order or position of observation contained by each class interval we need to find cumulative frequency. From the above table, now we know that observations 1st to 10th will contain class interval 0-10, observations 11th to 22nd will contain class interval 10-20, and so on.
Step 2: Identify the class in which median falls (median class is the class where n/2 lies, where n is the total number of observations)
NOTE :
n/2 = 50/2 = 25, so our median class is 20-30 because 25th observation lies in the class interval 𝑛
20-30.
In the class interval 20-30, we have 8 observations i.e., 23th , 24th, 25th, 26th, 27th, 28th, 29th and 30th.
We need to find the value of the 25th observation.
Step 3: How to find the value of 25th observation?
NOTE :
To find the value of 25th observation we follow the given procedure:
The class size of each class interval is 10, so we equally divide 10 in 8 equal parts.
10/8 = 1.25
The value of 23th observation is 20+1.25 = 21.25
The value of 24th observation is 20+2(1.25) = 20+ 2.50 = 22.5
Similarly the value of 25th observation is 20+ 3(1.25) = 20+ 3.75 = 23.75
Therefore, the median of the given group data is 23.75
1. Write the answer of the median calculated by you using ungrouped data.
2. Why do we get different values of median from grouped and ungrouped data?
Share your observations, reflections, questions and conclusions related to finding the median
when grouped data is given.
Derived formula for median is
Where l is the lower limit of the median class
n is number of observations
cf is the cumulative frequency of class preceding the median class.
f is the frequency of median class.
h is the class size.
Consumption of electricity
A colony's appropriate electricity consumption is being promoted by an NGO. He gathered
information on the electrical use of 150 colony families. The following frequency distribution
table shows the monthly consumption of electricity of 150 consumers of a locality.
1. What do you infer from the above data?
2. Which factors create differences in the electricity consumption of different families?
3. How do you plan an awareness drive in your area for adequate use of electricity?
4. Find the median of the given data.
Practice work
1. The salary (in rupees) of 45 employees of a Company is given below:
15000, 15000, 30000, 25000, 18000, 45000, 49000, 16000, 19000, 20000, 27000, 60000, 90000, 32000, 36000, 15000, 26000, 80000, 24000, 25000, 12000, 17000, 27000, 38000, 90000, 24000, 35000, 40000, 18000, 18000
20000, 24000, 29000, 40000, 23000, 25000, 60000, 90000, 23000, 100000, 27000, 15000, 24000, 20000, 18000
a) Find the median of the given data?
b) Share and discuss the possible methods to find the median of the given data.
c) Discuss which method is more convenient in finding the median in the given data.
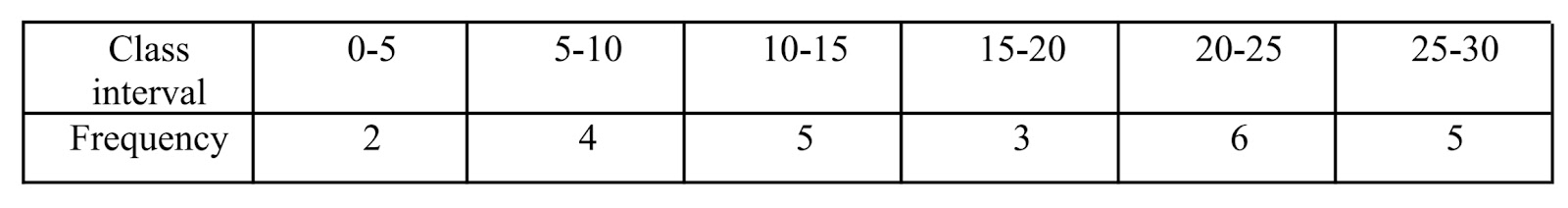
2. Find the median of the following frequency distribution table:
3. Find the median of the following frequency distribution table:


Post a Comment
Post a Comment