PROBABILITY
Task 12 : Energizer: Who will be the winner?
Number slips from 1 to 50 will be placed in a box. The game will be played between three
groups of students.
A group has to select one choice out of three given choices. (If two or all the students have same choice then toss or slips will be placed)
Choice 1: Getting a multiple of 10.
Choice 2: Getting a multiple of 2
Choice 3: Getting a multiple of 5.
This will be a 15 rounds game. The group who wins maximum points will be declared the winner of the game.
One slip is randomly selected from the box. If the slip is a multiple of 2 then group opted
multiple of 2 will award a point, If the slip is a multiple of 5 then group opted multiple of 5 will
award a point, If the slip is a multiple of 10 then group opted multiple of 10 will award a point
and If the slip is not a multiple of 2, 5 and 10 then no points will be awarded.
Record the winning points using tally marks:
● Is this game fair?
● Which player would you prefer to be and why?
● What changes could you make to the game to make it more fair?
● How does probability help you in finding the result or chance of happening any event?
Task 13 Theoretical probability
Cricket Match
In a cricket match, How does the empire decide which team bat or ball first?
Why did the Empire use coins to decide?
When the Empire tosses the coin, the captain of the team calls tail. What is his chance of winning the toss?
Is the chance of getting head or tail equally likely?
What is probability?
Who will bat first?
Imagine the situation when you are going to play cricket with your friends. How do you decide, who will bat first, second and so on?
Is your method fair? How?
Frame some probability questions from the Event and discuss the possible answers in the class?
NOTE :
A coin toss has only two possible outcomes: head or tail. Both outcomes are equally likely. This means that the theoretical probability to get either heads or tails is 0.5 or ½ (50%).
Theoretical probability of an outcome is based on mathematical analysis rather than physical analysis.
Think and discuss:
● Share your observations in your group.
● When you toss the coin 10 times, do you get five heads and five tails each time? Why or why
not?
NOTE :
In reality, your actual outcome may have been 3 heads and 7 tails or 4 heads and 6 tails or other.
These numbers would be your experimental probabilities. In reality, if you get 4 heads out of 10,
the experimental probability of head will be 4/10 or 0.4 and experimental probability of tail will
be 6/10 or 0.6.
Think and discuss:
● What is experimental probability?
● Do our experimental probabilities always match with calculated (theoretical) probabilities?
Justify.
NOTE :
Experimental probability is probability that is determined on the basis of the results of an
experiment repeated many times.
If you keep tossing the coin, you've probably noticed that the experimental probability got closer to the theoretical probability as you conducted more trials (coin tosses). Theoretical probability cannot tell you what will actually happen; it can only estimate the likelihood that an event will take place or that a particular result will occur.
Task 14 Experimental Probability
Activity 1:
Purpose: To find the change in the probability of an event when we increase the number of
trials.
Take any coin, toss it 100 times and note down the number of times a head and a tail comes up.
Record your observations at each step in the following table:
Fill the table using information given in table 1.
● Find the value towards which value of the fraction is approaching?
NOTE :
You will find that as the number of tosses gets larger, the values of the fractions come closer to 0.5 or ½.
Activity 2:
Purpose: To find the change in the probability of an event when we increase the number of
trials.
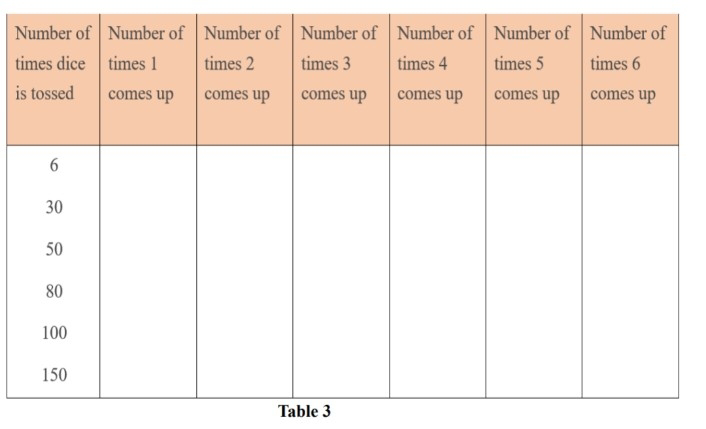
Take any dice, toss it 100 times and note down the number of times 1 or 2 or 3 or 4 or 5 or 6
comes up. Record your observations at each step in the following table:
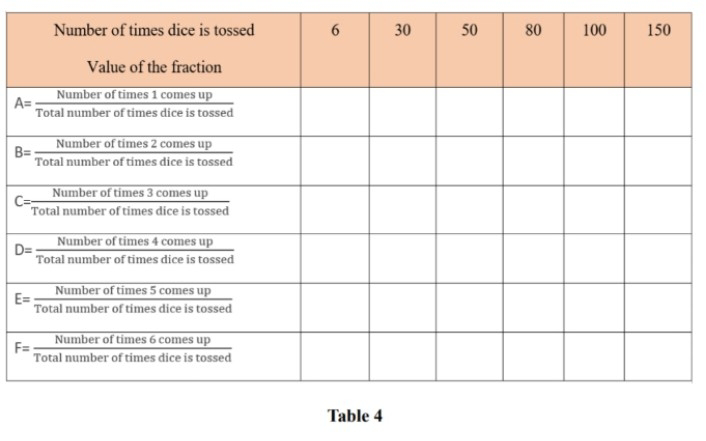
Fill table 4 using information given in table 3.
NOTE-
You will find that as the number of tosses gets larger, the values of each fractions come closer to 0.166 or 1/6.
Activity 3:
Purpose: To find the change in the probability of an event when we increase the number of
trials.
Toss two coins simultaneously 150 times and record your observations in the form of a table as given below:
Fill the table using information given in table 5
● Find the value towards which value of each fraction P, Q and R is approaching?
NOTE
You will find that as the number of tosses of two coins gets larger, the values of each fractions come closer to 1/4, 2/4 , ¼ respectively.
Think, pair and share:
Answer the following questions on the basis of your observations on the three activities:
● What is a trial?
● What is trial in activity 1, 2 and 3? Write two more examples.
● What are possible outcomes?
● What are possible outcomes in activity 1, 2 and 3? Write two more examples of a trial and
write their possible outcomes.
● What is an event?
● Write some possible events in activity 1, 2 and 3?
● What is probability?
● If you toss a coin, what are the number of possible outcomes? Are all the outcomes equally likely? Justify.
● If you toss a dice, what are the number of possible outcomes when the dice was not
manipulated? Are all the outcomes equally likely? Justify.
● Is picking a card from a well-shuffled deck of cards a random experiment? Justify your
answer.
● What is experimental or empirical probability?
● What is theoretical probability?
● What is the probability of an impossible event? Justify.
● What is the probability of a certain event? Justify.
● What is the range of probability of an event? Justify.
● What is the difference between theoretical and experimental probability?
NOTE:
In Activity 1, each toss of a coin is called a trial.
Similarly in Activity 2, each throw of a die is a trial, and each simultaneous toss of two coins in
Activity 3 is also a trial.
INFORMATION :
Trial- A trial is an action which results in one or several outcomes.
The possible outcomes in Activity 1 were Head and Tail, whereas in Activity 2, the possible
outcomes were 1, 2, 3, 4, 5 and 6 and possible outcomes in Activity 3 were HH, TT, TH and HT.
In Activity 1, the getting of a head in a particular throw is an event with outcome ‘head’.
Similarly, getting a tail is an event with outcome ‘tail’.
In Activity 2, the getting of a particular number, say 1, is an event with outcome 1. If our
experiment was to throw the die for getting an even number, then the event would consist of
three outcomes, namely, 2, 4 and 6.
INFORMATION :
● An event for an experiment is the collection of some outcomes of the experiment.
● The chance of happening of any event is expressed as a numerical value which is called
Probability.
Let n be the total number of trials. The empirical probability P(E) of an event E happening,
is given by
𝑁𝑢𝑚𝑏𝑒𝑟 𝑜𝑓 𝑡𝑟𝑖𝑎𝑙𝑠 𝑖𝑛 𝑤ℎ𝑖𝑐ℎ 𝑒𝑣𝑒𝑛𝑡 ℎ𝑎𝑝𝑝𝑒𝑛𝑒𝑑
P(E) = _____________________________________
𝑇𝑜𝑡𝑎𝑙 𝑛𝑢𝑚𝑏𝑒𝑟 𝑜𝑓 𝑡𝑟𝑖𝑎𝑙𝑠
In this chapter, we shall be finding the empirical probability, though we will write ‘probability’ for convenience.
The experimental probability depends on the number of trials undertaken, and the number of
times the outcomes you are looking for coming up in these trials.
Check your understanding:
1. Questions is based on results of activity 1:
(i) Find the probability of getting head in 10 tosses of a coin?
(ii) Find the probability of getting a head in 50 tosses of a coin?
(iii) Find the probability of getting a tail in 100 tosses of a coin?
2. Questions is based on results of activity 2:
(i) Find the probability of getting ‘6’ in 50 throw of a dice?
(ii) Find the probability of getting a ‘5’ in 80 throw of a dice?
(iii) Find the probability of getting a ‘2’ in 150 throw of a dice?
3. Questions is based on results of activity 3:
(i) Find the probability of getting one head in 20 tosses of a pair of coin?
(ii) Find the probability of getting a two head in 50 tosses of a pair of coin?
(iii) Find the probability of getting no head in 100 tosses of a pair of coin?
Group task: Case study
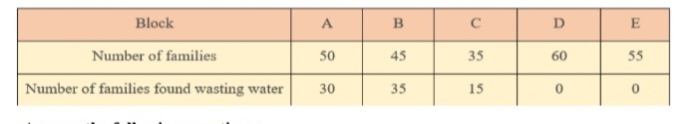
1. A colony consists of five blocks A, B, C, D and E. The number of families residing in each
block is given below:
All the block gets water facility by supply of water from Delhi Jal Board pipes. It was observed that many families waste the water by Car washing, street cleaning, floor washing, etc. Due to which Block D and E do not get proper supply of water.
A survey was done in this regard. The data collected through the survey is given below:
(a) What is the probability of water wasted by the block A families?
(b) What is the probability of water wasted by the block D families?
(c) What is the probability of water wasted by the block B and C families?
(d) Which block is less aware about the importance of water? Justify your answer.
(e) Are D and E block very much aware about the importance of water? Justify your answer.
(f) What measures you will take to stop the wastage of water in your area so that every
family get adequate water for the use?
Practice work
1. A coin is tossed 100 times with the following frequencies: Head: 60, Tail: 40
Compute the probability of each event?
2. Seven bags of wheat flour each marked 3 kg, actually contained the following weights of
flour in kg: 2.98, 3.05, 3.03, 3.00, 3.06, 2.96, 3.01;
Find the probability that any of these bags chosen at random contains more than 3 kg of
flour?
3. Three coins are tossed simultaneously 100 times. The following outcomes are recorded.
Find the probability of coming up more than one tail.
4. A die is thrown 300 times with the frequencies for the outcomes 1, 2, 3, 4, 5 and 6 as given in the following table :
(i) an odd number
(ii) a prime number
(iii) a number more than 1
(iv) a number multiple of 3.
5. A recent survey found that income of 150 workers in the Industrial area is as given
below:
(i) getting salary between 35000 to 45000
(ii) getting salary more than 45000
(iii) getting salary less than 25000.
6. In a particular session of class IX, 50 students were asked about their birth and following graph was prepared for the data, so obtained.
born in month of July.
7. The distance of 40 employees from their residence to the place of work is given in the table:
(i) Less than 10 km from his place of work.
(ii) More than 15 km from his place of work.
8. A tyre manufacturing company kept a record of the distance covered before a tyre needed
to be replaced. The table shows the results of 1000 cases.
hat is the probability that:
(i) it will need to be replaced before it has covered 20000 km?
(ii) it will last more than 30000 km?
9. In a cricket match, a batswoman hits a boundary 6 times out of 30 balls she plays. Find the probability that she did not hit a boundary.
10. Fifty seeds were selected at random from each of 5 bags of seeds, and were kept under
standardised conditions favourable to germination. After 20 days, the number of seeds which had germinated in each collection were counted and recorded as follows:
(i) more than 40 seeds in a bag?
(ii) 45 seeds in a bag?
(iii) less than 49 seeds in a bag?















Post a Comment
Post a Comment