Page: 50
1. Draw a rectangle of length 9 cm and width 6 cm. Divide it into three equal parts and complete the flag.

The top one-third of our flag is saffron (or orange).
(i) What is the colour of the middle one-third of the flag?
Sol. The colour of the middle one-third of the flag is white.
(ii) Where will you draw the Ashoka chakra?
Sol. I will draw the Ashoka chakra in middle of white portion of the flag.
(iii)How much of the flag will you colour green?
Sol. (1/3)rd portion of the flag will be coloured green.
(iv) Is the white colour now less than 1/3 of the flag? Why?
Sol. Yes, white colour is less than 1/3 of the flag. Because, Ashoka chakra is present in the white portion.
The Flag of Afghanistan
2. Now look at this flag. How much of it is Black?
Sol. There are 3 portion, so (1/3)rd portion of the flag contains black colour.
(i) The green part of the flag can be written as .
Sol. The green part of the flag can be written as one-third.
(ii) Is red less than one-third of the flag? Why?
Sol.
Yes, red colour is less than 1/3 of the flag. Because, white emblem is present in the red portion.
3. This is the flag of Myanmar, our neighbor.

(i) Is blue more than one-fourth of the flag or less?
Sol. Blue colour is less than one-fourth of flag.
(ii) Guess how much of the flag is red. Is it more than ½? Is it more than three-fourths?
Sol. It is more than half and also it is more than three-fourths.
Find Out
1.Collect as many flags as you can.
How many flags have three colours? Are all the coloured parts equal in these flags?
Ans.
USA
Malaysia
Azerbaizan
United kingdom
Venuzuela
Ghana
Flags of Ghana, Azerbaizan, Malaysia and Venezuela have three colours.
2.This is the flag of the Math Club in a school in Kerala. What part of the flag is coloured red? What part is green?
Ans. Two-fifth part of the flag is in red colour. One-fifth part of the flag is in green colour.
3.![]()
Ans.We can draw the black logo as under:![]()
4.Is there a Math Club in your school? If not, ask your teacher how to set it up. Design a flag for your Math Club. Draw it here.
Ans. No, there is no Math Club in my school. I have requested my teacher to help us in setting up a Math Club. I have designed the following flag for our math club.
5.Have you used the red colour? What part of the flag did you colour red? What were the other colours you choose?
Ans. Yes, I have used the red colour. The logo is made in red colour. Apart from red, I have used orange, sky blue and green colours in the flag.
Magic Top
Let us make a magic top.
Take a cardboard piece.
Draw a circle of radius 3 cm and cut it out.
Divide the circle into 8 equal parts. Now each part is 1/8 of the circle.
Colour 2/8 red, 1/8 orange, 1/8 yellow, etc. as shown here. Push a matchstick through the centre of the circle.Your magic top is ready Spin it fast!
1.What do you see? Can you see all the colours? Write, what you see in your notebook.
Ans. When the magic top is spinning, we cannot see the colours properly. We see a white circular top spinning.
4. Practice time
(A) Chocolate bar

Manju had a chocolate. She gave one-fourth of it to Raji, one-third to Sugatha and one-sixth to Sheela. She ate the remaining part. How many pieces of chocolate did each get? Write here.
Sol.
Manju had a chocolate.
Number of bites in the chocolate is 12.
She gave one-fourth of it to raji = ¼ × 12
= 3 bites
Sugatha got one-third of it = (1/3) × 12
= 4 bites
Sheela got one-sixth of it = (1/6) × 12
= 2 bites
(i) What part of the chocolate did Manju eat?
Sol.
Part of the chocolate remains with manju = 12 – (3 + 4 + 2)
= 12 – 9
= 3 bites
Therefore, Manju eat 3 bites of chocolate.
(B) Colour the hats

(i) Colour 1/3 of the hats red.
(ii) Colour three-fifth hats blue.
(iii) How many hats did you colour red?
(iv) How many hats did you colour blue?
(v) What part of the hats are not coloured?
Sol.
The total number of hats in the given figure = 15
(i) Out of 15 colour 1/3 of the hats red = 1/3 × 15
= 5 hats
(ii) Out of 15 colour three-fifth hats blue = 3/5 × 15
= 3 × 3
= 9 hats
(iii) 5 hats are coloured red.
(iv) 9 hats are coloured blue.
(v) Number of hats coloured = 14
Total number of hats = 15
So, Number of hats not coloured = 15 – 14
= 1 hat
Therefore, (1/15)th part of the hats are not coloured.
Equal Parts of a Triangle
1.The white triangle is divided into three equal parts. Fill each one-third part with a different colour. Can you show that these parts are equal? Think how.
Ans. Yes, this can be done by making a cut of the parts of the triangle by using tracing paper.
If one part is placed over another part, it is seen that the first part fully covers the second part. Moreover, the second part also fully covers the first part or the third part. This shows that all parts are equal.
2.Now try to make three equal parts of this triangle in a different way. Colour each one-third with a different colour.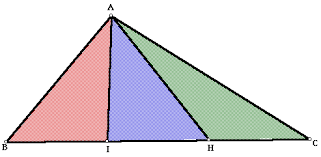
Ans. The given triangle is coloured with different colours,
This is shown in the following figure.
Page 54
Six parts of a Rectangle
Rani has divided a green rectangle into six equal parts like this.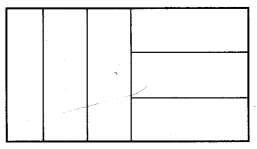
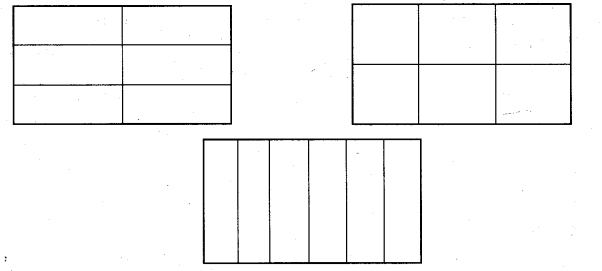
1.Now you divide each of these rectangles into six equal parts. Use a different way for each of the three rectangles.
Ans. These rectangles can be divided into six equal parts as follows:
Young Birbal agreed since he had no other way.
When he went in, the gatekeeper calculated "If he gets 100 gold coins I will get_10_gold coins".
The poet came to a second gatekeeper.
This gatekeeper also said. "I will let you in if you give me two-fifth of your prize". The poet agreed.
The gatekeeper happily calculated. "The poet will get at least 100 gold coins so I will get 40 gold coins!"
The poet reached the last gate. The gatekeeper said. "I will allow you to see the king only if you give me half of the prize that you get". The poet had no other way. He agreed and went inside.
The gatekeeper thought. "Today is a great day. If he gets 100 gold coins I will get __1/2 of 100 = 50____ gold coins. But if he gets 1000 coins - wow! I will get __1/2 of 1000 = 500.
The king was very happy with the poems and said, "Your work is very good. You can ask anything as your prize".
"My Lord, I want 100 slaps". "What! 100 slaps?
was shocked_ ". The king
* What happened after that? Complete the story. What part of the prize did the poet get?
Ans. The gatekeeper at out gate got 10 slaps, the gatekeeper at second gate got 40 slaps and the gatekeeper at the last gate got 50 slaps. The poet got zero slaps, i.e, no slaps.
Patterns in parts Page: 57
1) Make different patterns by colouring some squares in the grids B, C, D. What part of the grid did you colour? What part of the grid remained white? Write.
Sol.

2) Look at grid A again. Is the grid coloured—
(a) 1/2 blue, 1/2 white?
(b) 2/4 blue, 2/4 white?
(c) 3/8 blue, 5/8 white?
(d) 4/8 blue, 4/8 white?
Ans. (c) is the wrong answer
Mark (X) on the wrong answer.
Sol.
(a) 1/2 blue, 1/2 white
(b) 2/4 blue, 2/4 white
(c) 3/8 blue, 5/8 white (X)
(d) 4/8 blue, 4/8 white
Because,
(a) 1/2 blue, 1/2 white = 8/16 = 1/2 … [divide both denominator and numerator by 8]
(b) 2/4 blue, 2/4 white = 8/16 = 2/8 … [divide both denominator and numerator by 4]
(d) 4/8 blue, 4/8 white = 8/16 = 4/8 … [divide both denominator and numerator by 2]
3) Draw grids of 16 squares and make patterns with
(a) 2/8 red, 1/2 yellow, 1/4 green
(b) 3/16 blue, 5/16 red, 1/2 green
Sol.
(a) 2/8 red, 1/2 yellow, 1/4 green
Out of 16 squares 2/8 red = 2/8 × 16
= 2 × 2 = 4 red squares
Out of 16 squares 1/2 yellow = ½ × 16
= 1 × 8 = 8 yellow squares
Out of 16 squares 1/4 green = 1/4 × 16
= 1 × 4 = 4 green squares

(b) 3/16 blue, 5/16 red, 1/2 green
Out of 16 squares 3/16 blue = 3/16 × 16
= 3 × 1= 3 blue squares
Out of 16 squares 5/16 red = 5/16 × 16
= 5 × 1 = 5 red squares
Out of 16 squares 1/2 green = 1/2 × 16
= 1 × 8 = 8 green squares

Ramu’s vegetable field Page: 58
Ramu’s vegetable field has 9 equal parts.
1. What vegetables does he grow?
Sol.
Ramu grows chillis, potatoes, brinjals, tomatoes and spinach in his vegetable field.

i) Which vegetable grows in the biggest part of his field? What part?
Sol.
He grows tomato in the biggest part of the field.
Out of 9 equal parts 3 of them contains tomato.
So (3/9)th part of his field contains tomato.
ii) On what part of the field does he grow potatoes?
Sol.
Out of 9 equal parts 2 of them contains potato.
So, (2/9)th part of his field contains potato.
iii) What part of the field is used to grow spinach? What part is used for brinjals?
Sol.
Out of 9 equal parts only one part contains spinach.
So, (1/9)th part of his field contains spinach.
Out of 9 equal parts 2 parts contains brinjals.
So, (2/9)th part of his field contains brinjals.
2. Ramu wanted to give these vegetables to his friends. He gave Aboobacker one-fifth of these tomatoes and 1/3 of the potatoes. Srija got 2/5 of the tomatoes and 3/6 of the potatoes. Nancy got the rest of these vegetables. Circle Aboobacker’s share in blue. Circle Srija’s share in yellow.

Sol.
From the given figure,
Total number of potatoes = 18
Total number of tomatoes = 20
From the question,
Ramu wanted to give these vegetables to his friends.
Aboobacker got one-fifth of these tomatoes
= (1/5) × 20 = 1 × 4 = 4
Aboobacker got 1/3 of the potatoes
= (1/3) × 18 = 1 × 6 = 6
Srija got 2/5 of the tomatoes
= (2/5) × 20 = 2 × 4 = 8
Srija got 2/5 of the Potatoes
= (3/6) × 18 = 3 × 3 = 9
Then,
Nancy got the rest of these vegetables.
Total number of vegetables including potatoes and tomatoes
= 20 + 18 = 38
Number of vegetables got by Aboobacker and Srija = 4 + 6 + 8 + 9
= 27
So, Nancy got = 38 – 27
= 11 vegetables.

(i) How many potatoes and tomatoes did Nancy get?
Sol.
From the above solution,
Total number of tomatoes = 20
Total number of potatoes = 18
Then,
Number of tomatoes got by Aboobacker and Srija = 4 + 8
= 12
Number of potatoes got by Aboobacker and Srija = 6 + 9
= 15
Now,
Number of tomatoes got by Nancy = 20 – 12
= 8 tomatoes
Number of potatoes got by Nancy = 18 – 15
= 3
Guess and Check Page: 61
A) What part of each shape is coloured?
First guess the answer, then check.

Sol.
By looking at the figure (1) we can say that, 1/8 of the shape is coloured.
(2)

Sol.

By looking at the figure (2) we can say that, 1/6 of the shape is coloured.
(3)

Sol.

By looking at the figure (3) we can say that, 2/9 of the shape is coloured.
(4)

Sol.

By looking at the figure (4) we can say that, 3/12 of the shape is coloured.
2. Coloured Parts
Complete these
(i) This circle is divided into two equal parts. Out of equal parts one part is coloured blue.
Sol.
This circle is divided into two equal parts. Out of equal 2 parts one part is coloured blue.
(ii) Here the circle is divided into equal parts. Out of equal parts, parts are coloured blue.
Sol.
Here the circle is divided into four equal parts. Out of four equal parts, parts two are coloured blue.
(iii) Here the circle is ………… …… .
Sol.
Here the circle is divided into six equal parts. Out of six equal parts, parts three are coloured blue.
(iv) Here the circle is …… … …… … …
Sol.
Here the circle is divided into eight equal parts. Out of eight equal parts, parts four are coloured blue.
So we can say that 1/2 = (2/4) = (3/6) = (4/8)
3. Cutting the Halwa
Ramesh bought a piece of halwa for his children Ammu and Anu.

He divided it equally for them.
(i) Each will get ________ part of halwa.
Sol.
Each will get ½ part of halwa.

This piece is too big. We can’t eat it, they said.
(ii) So he divided the pieces into half again. Now how many pieces will Ammu get?
Sol.
Ammu get two pieces.

(iii) What part of the halwa is it?
Sol.
2/4 part of halwa, or ½ part of halwa.
“Make it even smaller, Dad” they asked.
So he again cut the halwa into smaller pieces.

“Ok, thank you, Dad”.
(i) Now how many pieces will each get?
Sol.
Now, Ammu and Anu get four piece each.
(ii) What part of the halwa is each piece now?
Sol.
Each piece is now 1/8 part of halwa.
(iii) If Ramesh had cut the halwa into 6 equal parts how many pieces each have got? Look at your answers for questions 1 to 4 and write — ½ = = =
Sol.
If Ramesh had cut the halwa into 6 equal parts each got 3 pieces of halwa.
Then,
½ = 2/4 = 3/6 = 4/8 = 5/10 = 6/12
4. Parts of the Strip
(i) Look at the picture. Write what part of the strip is each green piece. Write the part for a piece of each colour.

Sol.

(ii) How many one-fourths will make a half?
Sol.
Two one-fourth will make a half.
= ¼ + ¼
= 2/4 [divide both numerator and denominator by 2]
= ½ = half
(iii) How many 1/8 will make ¼?
Sol.
Two 1/8 will make ¼.
= 1/8 + 1/8
= 2/8 [divide both numerator and denominator by 2]
= ¼
(iv) How many 1/8 are in ½?
Sol.
Four 1/8 are in ½.
= 1/8 + 1/8 + 1/8 + 1/8
= 4/8
= ½ [divide both numerator and denominator by 4]
5. Patterns
Look at the square.

(i) What part is coloured blue?
Sol.

From the above figure, we can say that, out of 16 squares 2 squares are coloured blue.
i.e. 2/16 or 1/8 part is coloured blue.
(ii) What part is green?
Sol.
From the above figure, we can say that, out of 16 squares 1 square coloured green.
i.e. 1/16 part is coloured green.
6. From a Part to the Whole
(i) This show 1/5 petals of a flower. Complete the flower by drawing the other petals.

Sol.

(ii) The picture shows one-third of the blades of a fan. Complete the picture by drawing the other blades.

Sol.

(iii) Half of the blades of another fan are shown here. Complete the picture by drawing the other half. How many blades have you drawn?

Sol.

7. Rupees and Paise

(i) How many will make one rupee?
Sol.
Two 50 paise coin make one rupee coin.
(ii) Is 50 paise half of one rupee?
Sol.
Yes, 50 paise is half of one rupee.

(iii) How many will make one rupee?
Sol.
Four 25 paise coin make one rupee.
(iv) 25 paise is _________ part of one rupee
Sol.
25 paise is ¼ part of one rupee.
(v) 20 paise is _________ part of one rupee
Sol.
20 paise is 1/5 part of one rupee.
Five 20 paise coin make one rupee.
(vi) How many 10 paise will make one rupee?
Sol.
Ten 10 paise coin make one rupee.
(vii) So 10 paise is _____ part of one rupee.
Sol.
So 10 paise is 1/10 part of one rupee.
8. Arun’s Time Table

Sleeping: One third of a day
Use different colours to show,
Playing: One eighth of a day
Studying: ¼ of a day
Sol.
We know that, 24 hours in a day and above figure contains 25 box.
So, Arun spent one eighth of a day for playing = (1/8) × 24
= 1 × 3
= 3 hours = 3 box
He spent ¼ of a day for studying = ¼ × 24
= 1 × 6
= 6 hours = 6 box

Sleeping Playing Studying Other activities
(i). How many hours does Arun take for Sleeping, studying and Playing?
Sol.
8 hours for sleeping, 6 hours for studying and 3 hours for playing.
(ii) What part of the day does he use for other activities?
Sol.
He use 7/24 part of the day for other activities.
9. School Magazine
A school has decided to bring out a magazine every quarter of the year. How many magazines will they have in a year? If they want to print it at the end of each quarter of a year, which are the months for printing? Mark the number for those months.

Sol.
From the question it is given that, school has decided to bring out a magazine every quarter of the year.
We know that, there are 12 months in a year.
Quarter of the year = ¼ × 12
= 1 × 3
= 3 months
So, for every three months, school has to bring out a new magazine.

10. Sleeping Beauty
Have you heard of Kumbhakarna, the brother of Ravana? He is famous for sleeping for half a year.
(i) Most people sleep about 8 hours a day. Then what part of a day is it?
Sol.
We know that, 24 hours in a day.
Given, most people sleep about 8 hours a day = 8/24
= 1/3 part of a day
(ii) So what part of a year do they sleep? A person 60 years old must have slept _______ years!!!
Sol.
A person 60 years old must have slept for = 1/3 × 60
= 1 × 20
= 20 years
11. Keerti’s shopping list

Look at the yellow price list.
a) How much does 2 kg of tomato cost?
Sol.
From the price list 1 kg of tomato = ₹ 12
Then, cost of 2 kg of tomato = 12 × 2
= ₹ 24
b) How much does kg of tomato cost?
Sol.
From the price list 1 kg of tomato = ₹ 12
c) Kiran wants 2½ kg of tomato. How much will it cost?
Sol.
From the price list 1 kg of tomato = ₹ 12
For ½ kg of tomato = ½ × 12
= ₹ 6
Then, cost of 2 kg of tomato = 12 × 2
= ₹ 24
So, cost of 2½ kg of tomato = 24 + 6
= ₹ 30
d) How much does 3½ kg potato cost?
Sol.
From the price list 1 kg of potato = ₹ 10
For ½ kg of potato = ½ × 10
= ₹ 5
Then, 3 kg of potato = 3 × 10
= ₹ 30
So, cost of 3½ kg of potato = 30 + 5
= ₹ 35
e) What is the price of 1¼ kg of carrot?
Sol.
From the price list 1 kg of Carrot = ₹ 18
For ¼ kg of carrot = ¼ × 18
= 9/2
= ₹ 4.5
Then, 1¼ kg of carrot = 18 + 4.5
= ₹ 22.5
f) He bought a gourd of weight 4¾ kg and it costs?
Sol.
From the price list 1 kg of Gourd = ₹ 8
For 4 kg of Gourd = 8 × 4
= ₹ 32
For ¾ kg of Gourd = ¾ × 8
= 3 × 2
= ₹ 6
Then, cost of 4¾ kg of Gourd = 32 + 6
= ₹ 38
g) Look at the shopping list in Keerti’s hand. How much will she have to pay to buy all of these?
Sol.
| Item | Price per kg | Total price |
| Potato 2¼ kg | 10 | 22.50 |
| Carrot 3¼ kg | 18 | 67.50 |
| Gourd 1 ½ kg | 8 | 12 |
| Total | ₹ 102 | |
12. Raheem’s journey
Raheem has to travel 1¼ km to reach school. What distance does he travel to go to school and come back home?
Sol.
From the question it is given that,
To reach the school Raheem has to travel = 1 ¼ km = 5/4 km
From school to home = 1 ¼ km = 5/4 km
Total distance travelled by Raheem = 5/4 + 5/4
= 10/4
= 5/2
= 2 ½ km
Therefore, he travel to go to school and come back home = 2 ½ km
13. What coins?
Latha bought a pencil and a pen for seven and a half rupees. She gave Rs 10/–. The shopkeeper gave back the money in half and quarter rupees. What are the coins she got?
Sol.
From the question it is given that,
Latha bought a pencil and a pen for = ₹ 7½
Money given by latha to the shopkeeper = ₹ 10
Shopkeeper gave back the money = 10 – 7.5
= ₹ 2.5
So, the shopkeeper can return as ₹ 2.5 in the following ways
a) 1 half rupee coin and 8 quarter rupee coins
b) 2 half rupee coins and 6 quarter rupee coins
c) 3 half rupee coins and 4 quarter rupee coins
d) 4 half rupee coins and 2 quarter rupee coins
14. At the railway station
Your attention please. Mangalore Express coming from Mangalore and going to Thiruvananthapuram is now running late by half an hour.
Oh the train is late today. The right time is a quarter to 7.
a) What time is the train expected to come today?
Sol.
The right time is a quarter to 7 i.e. 6: 45
But, train is delayed by half an hour i.e. 30 minutes.
So, exact time train will arrive at 6: 45 + 0: 30 = 7: 15
b) Nazia gets off at a station after 2½ hours from this station. What time will she get off?
Sol.
From the question,
Nazia gets off at a station after = 2: 30 hours
Then, total time taken by Nazia to reach = 2: 30 + 7: 15
= 9: 45
c) Shaji will take 5 hours to reach Ernakulam by this train. At what time will he reach there?
Sol.
From the question, it is given that,
Shaji will take 5 hours to reach Ernakulam
Then, total time taken by Shaji to reach Ernakulam = 7: 15 + 5
= 12: 15






































Post a Comment
Post a Comment